SAT Math Level I-II. Problem solving & SAT Math. Kalomitsenes – Travlopanos
€28.00 συμπερ. Φ.Π.Α.
SPECIAL OFFER FOR ABROAD (ALL OVER THE WORLD) No extra charge for shipping worldwide (regardless of order amount)
payment for all countries only with credit card (except of shipment inside Greece)
Τρόπος πληρωμής μόνο για αποστολές εντός Ελλάδας
- Αντικαταβολή (πληρώνετε μετρητά στον ταχυδρόμο δίχως επιπλέον χρέωση)
- Όλες οι πιστωτικές κάρτες (δίχως επιπλέον χρέωση),
- Tραπεζικό έμβασμα e banking (δίχως επιπλέον χρέωση
 SAT Math Level I-II. Problem solving & SAT Math.
SAT Math Level I-II. Problem solving & SAT Math.
Spyros Kalomitsines & Fotios I. Travlopanos, 24grammata ed
ΖΗΤΗΣΤΕ ΤΑ ΣΕ ΟΛΑ ΤΑ ΒΙΒΛΙΟΠΩΛΕΙΑ
και ONLINE https://24grammata.com/product/8256/
SPECIAL OFFER FOR ABROAD (ALL OVER THE WORLD) No extra charge for shipping worldwide (regardless of order amount)
payment for all countries only with credit card (except of shipment inside Greece)
ΔΩΡΕΑΝ ΤΑΧΥΔΡΟΜΙΚΑ για όλη την επικράτεια,
καθώς και τηλεφωνικά στο 210 612 70 74 / αντικαταβολή / ΔΩΡΕΑΝ ΤΑΧΥΔΡΟΜΙΚΑ για όλη την επικράτεια, ανεξάρτητα του ποσού παραγγελίας
see also, Problem Solving – Effective methods for High School
and University students – How to become better problem solvers
ONLINE https://24grammata.com/product/3256/
To the Reader
We believe that the present work should help considerably the students to their maths examinations, either for the mathematical competitions as well as in SAT math Level 2 exams.
In case you are going to take the SAT math Level 2 examinations, then:
• 1. Read some pages from the first part of this book. You will learn techniques on How you can help your mind generate more ideas in problem solving.
Then go to the other part.
• 2. Here, there is a selection of problems for SAT Mathematics examinations, from all chapters.
The solutions are presented in a very easy way, using simple and effective techniques.
• 3. We apply, in a very simple and instructive way, knowledge from Cognitive Psychology (see: How the mind works, 1980) which is the easiest and fastest way to learn and remember. In few days, or in two weeks, you can go through this point, and then you will gain
much self – confidence in solving problems.
• 4. If you already have any textbook, use it parallel to this part. You will very soon be able to achieve a high score in the examinations. The more you practise, the greater the speed of the brain increases. Mathematics will be very easy for you, it will be a
pleasure . . .
Title:SAT Math Level I-II. Problem solving & SAT Math.
Writers: Spyros Kalomitsines & Fotios I. Travlopanos
ISBN: 978-618-2010-92-5
Pages: 344
Publishing House: 24grammata
77 Pentelis Avenue, Chalandri, 152 34, Athens, Greece
Τelephone: +30 210 612 70 74
Email: [email protected]
Web site / e-shop: www. 24grammata.com
Copyright © 2020 24grammata
Book
Contents
1 Introduction 1
1.1 Technique A . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 1
1.2 More applications of Technique A . . . . . . . . . . . . . . . . . . . . . . . . 5
1.3 Categories & a longer discussion on short notes . . . . . . . . . . . . . . . . 9
1.4 Applications – Problems . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 11
1.5 Technique B – How to get out of loops . . . . . . . . . . . . . . . . . . . . . 17
1.6 A few applications to problems for mathematical competitions . . . . . . . . 20
2 Topic 1 – Arithmetic-Algebra & Geometry 35
2.1 Problems on percentages . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 36
2.2 Ratio & Proportion . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 43
2.2.1 Examples & Techniques for problems on directly, or, inversely propor-
tional values . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 46
2.3 Inverse, odd-even & Combining functions . . . . . . . . . . . . . . . . . . . . 48
2.3.1 Odd – Even Functions . . . . . . . . . . . . . . . . . . . . . . . . . . 51
2.3.2 Combining functions & inverse functions . . . . . . . . . . . . . . . . 53
2.4 Absolute value & Inequalities . . . . . . . . . . . . . . . . . . . . . . . . . . 57
2.5 Geometry & Formulas . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 71
2.5.1 A summary of geometric formulas . . . . . . . . . . . . . . . . . . . . 71
2.5.2 Geometry Examples & Problems . . . . . . . . . . . . . . . . . . . . 75
3 Topic 2 – Functions 91
3.1 Linear Functions . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 91
3.1.1 General form of the equation . . . . . . . . . . . . . . . . . . . . . . . 92
3.1.2 Slope formula . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 92
3.1.3 Parallel lines . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 92
3.1.4 Perpendicular lines . . . . . . . . . . . . . . . . . . . . . . . . . . . . 93
3.1.5 Midpoint & Distance of points . . . . . . . . . . . . . . . . . . . . . . 93
3.1.6 Distance of a point from a straight line . . . . . . . . . . . . . . . . . 93
3.1.7 Angle . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 94
3.2 Examples of Linear functions & graphs . . . . . . . . . . . . . . . . . . . . . 94
3.2.1 Multiple Choice Problems . . . . . . . . . . . . . . . . . . . . . . . . 98
3.3 Quadratic functions . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 108
3.3.1 Problems on quadratics . . . . . . . . . . . . . . . . . . . . . . . . . . 110
3.4 Word Problems . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 120
3.5 Polynomial Functions . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 123
3.5.1 The remainder & factor theorems . . . . . . . . . . . . . . . . . . . . 123
3.5.2 Problems on the remainder theorem . . . . . . . . . . . . . . . . . . . 124
3.6 Rational Functions & Limits . . . . . . . . . . . . . . . . . . . . . . . . . . . 133
3.6.1 Domains of functions . . . . . . . . . . . . . . . . . . . . . . . . . . . 133
3.6.2 How to find limits – 2 mnemonic rules . . . . . . . . . . . . . . . . . . 142
3.7 Exponential & Logarithmic function . . . . . . . . . . . . . . . . . . . . . . 150
3.7.1 Summary . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 150
3.7.2 Logarithms . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 151
3.7.3 Problem Solving now . . . . . . . . . . . . . . . . . . . . . . . . . . . 152
3.8 Parametric Equations . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 161
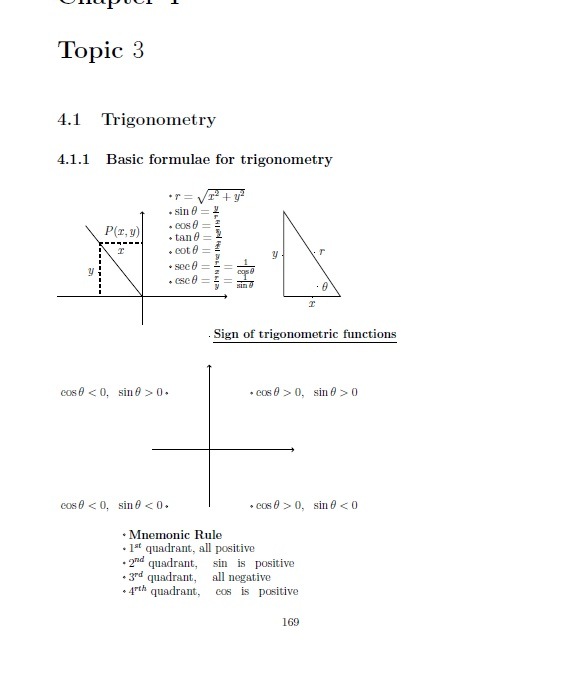
4 Topic 3 169
4.1 Trigonometry . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 169
4.1.1 Basic formulae for trigonometry . . . . . . . . . . . . . . . . . . . . . 169
4.1.2 Examples & problems for trigonometry . . . . . . . . . . . . . . . . . 172
4.2 Analytic & Coordinate Geometry . . . . . . . . . . . . . . . . . . . . . . . . 186
4.2.1 Basic equations – Analytic Geometry . . . . . . . . . . . . . . . . . . 186
4.2.2 Problems on 2 dimensional coordinate plane . . . . . . . . . . . . . . 188
4.2.3 Polar Coordinates in plane geometry . . . . . . . . . . . . . . . . . . 201
4.2.4 3 – dimensional geometry . . . . . . . . . . . . . . . . . . . . . . . . . 203
5 Topic 4 – Sequences – Complex numbers – Vectors – Matrices 207
5.1 Sequences . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 207
5.1.1 Summary . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 207
5.1.2 Arithmetic sequences . . . . . . . . . . . . . . . . . . . . . . . . . . . 207
5.1.3 Geometric sequences or Progressions . . . . . . . . . . . . . . . . . . 213
5.1.4 Multiple Choice Problems . . . . . . . . . . . . . . . . . . . . . . . . 215
5.2 Complex Numbers . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 219
5.2.1 Basic theory . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 219
5.3 Vectors . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 228
5.3.1 Basic theory on vectors . . . . . . . . . . . . . . . . . . . . . . . . . . 228
5.3.2 Examples & problems on vectors . . . . . . . . . . . . . . . . . . . . 228
5.4 Matrices . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 241
5.4.1 Problems . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 244
6 Topic 5 – Data Analysis – Combining & Probabilities 253
6.1 Statistics . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 253
6.2 Probability . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 257
6.2.1 Mutually exclusive events . . . . . . . . . . . . . . . . . . . . . . . . 258
6.3 Some practice now . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 259
6.4 Counting rule . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 263
6.5 Sample Space & Probabilities . . . . . . . . . . . . . . . . . . . . . . . . . . 274
7 Practice Tests 285
7.1 Practice Test A . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 285
7.1.1 Solutions of Practice Test A . . . . . . . . . . . . . . . . . . . . . . . 292
7.2 Practice Test B . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 305
7.2.1 Solutions of Practice Test B . . . . . . . . . . . . . . . . . . . . . . . 312
Appendices 327
Contents
A To the Editors 329
A.1 Comments regarding Kalomitsines’ ideas . . . . . . . . . . . . . . . . . . . . 329
Appendix A
To the Editors
A.1 Comments regarding Kalomitsines’ ideas
• a. W. Wickelgren – he has worked on problem solving at the MIT and he has written
a book on problem solving. After reading the technique wrote to Mr. Kalomitsines:
. . . Let me emphasize that I believe your ideas are ingenious and of great value in im-
proving peoples problem solving. The students of the world will be the losers if you
are too modest to publish your ideas in prominent outlets . . .
Note:
After the suggestion of professor J. Greeno in 1980 I made some computer programs in
LRDC of Pittsburgh University, using methods and concepts of Cognitive Psychology
which I was taught at the Carnegie – Mellon University by H. Simon (Nobel 1978).
The computer solved a number of difficult problems from Euclidean Geometry and
Group Theory. I was there as a Fullbright Scholar. Then J. Greeno wrote:
• a. J. Greeno – University of Pittsburgh and Stanford University:
(i) . . .The progress made in developing new theoretical analysis was much greater
than any I have ever observed, due to Mr. Kalomitsines very intensive efforts and the
thoroughness of the thinking he has previously done, on the topic of problem solving
. . .
ii Many of us hope that the concepts and methods of cognitive psychology can
be used profitably, by teachers and other developers of educational materials, to help
provide clear and definite expressions of their ideas and methods. Kalomitsine’s report
gives an example that provides justification for this hope.
• c. A. Posamentier – Journal: The Mathematics Teacher
. . . the instruction of Mathematics would be greatly strengthened if every Mathematics
teacher would incorporate the many valuable ideas presented in this little book . . .
These comments refer to a book by the author, entitled Attack your problem. Read
also a review from Mathematical Association of America (MAA) (by David
A. Hackaby, on 03/31/2009) for Kalomitsines’ book: How to solve problems:
New methods and Ideas.
An extract from this review is: Anyone who applies these methods to solve the many
examples and problems in this book cannot help but become a better problem solver.
329
Some more comments:
Professor Melas A., a talented mathematician, gold medalist in two Mathematical
Olympiads, who is currently a celebrated mathematician at the University of Athens,
when first read the technique, said:
This is the way I think when I solve problems.
An Olympiad medalist in Mathematics – once said to Spyros Kalomitsines:
I had gotten stuck with a problem in Functional Analysis. Then I decided to try your
technique and I found the solution easily. This technique means to me: Pat the
problem and it will respond!
Applications of the methods to other areas:
1) G. Leondiadis, a researcher in bio-medicine in Canada, sent an e-mail to Mr.
Kalomitsines
. . .Your methods hooked me. They are ingenious, especially the How to get out of
loops. I am sure they will be helpful in my research.
2) The late H. Simon (Nobel 1978) researcher in Cognitive Psychology said to Mr.
Kalomitsines the technique of getting out of loops is very interesting.
• Searching the internet:
This book is unique in that it describes the actual method of problem solving. This is the
classic book on problem solving.
• Some comments on Spyros Kalomitsines research at the LRDC of Pitts-
burgh University:
. . .we greatly admire the results of your work here . . . , J. Greeno, 5 December 1980
(professor at Pittsburgh University and Stanford later)
• Professor Steve Krantz (May 18, 2016):
. . . quite interesting and different from anything else I have seen. (e-mail to Sp. Kalomitsines)
• Professor D. Bergeles, first winner in a difficult mathematical competition,
(November 10, 2014 ):
. . .when we try to solve a difficult problem, Mr. Kalomitsines managed to describe, ex-
plicitely and succesfully, the steps that very often and unconciously we follow, trying to
surpass dead ends or trying to get out of any loops . . .
330
Related products
Ε16. εκπαίδευση
Ε.37 Διαγωνισμοί Δημ. / Κατατακτ.
Σύγχρονο περιβάλλον γραφείου, Digital Office, από τα Λογιστικά Φύλλα στο Διαδίκτυο
Ε16. εκπαίδευση
Ε16. εκπαίδευση
Ε.37 Διαγωνισμοί Δημ. / Κατατακτ.
Μηχανογραφημένη Λογιστική (Έξυπνος οδηγός για Γρήγορη Εκμάθηση + CD) Χαρά Ν. Κοτταρά
E30. nautical pulications
Στοιχεία Ναυτικής Μετεωρολογίας, Γεώργιος Τσιμίνος, Πλοίαρχος Ε.Ν.
Ε16. εκπαίδευση
Χημεία Γ΄ Λυκείου Επαναληπτικά Θέματα και Κριτήρια Αξιολόγησης, Μάριος Τριανταφύλλου
E30. nautical pulications
Ε16. εκπαίδευση
Ε16. εκπαίδευση
ΗΛΕΚΤΡΟΛΟΓΩΝ ΕΙΔΙΚΟΤΗΤΑΣ Α’ : Φωτισμού, Κίνησης & Παραγωγής / Διανομής Ενέργειας πρακτικό μέρος
E30. nautical pulications
Ε16. εκπαίδευση
Ε16. εκπαίδευση
Ε16. εκπαίδευση
Ε16. εκπαίδευση
Ε16. εκπαίδευση
The Olympic mathematic marathon grades 7-12 volume 1, Γιώργος Αποστολόπουλος, Ντανιέλ Σιτάρου
E30. nautical pulications
E30. nautical pulications
Ε16. εκπαίδευση
E30. nautical pulications
Ε16. εκπαίδευση
Ε16. εκπαίδευση
Ε16. εκπαίδευση
Ε16. εκπαίδευση
Ε16. εκπαίδευση
Ε16. εκπαίδευση
Ε16. εκπαίδευση
Ε.37 Διαγωνισμοί Δημ. / Κατατακτ.
Ε16. εκπαίδευση
Συμμετέχοντας στη… σύν«ταξη» για όλο το Δημοτικό Ηρακλής Αρ. Καρακόλιος, Νικόλαος Αθ. Σφυρής
Ε16. εκπαίδευση
Ε16. εκπαίδευση
Τράπεζα Θεμάτων Φυσικής Β΄ Λυκείου Κατεύθυνσης (Μάιος 2022) Επιμέλεια: Ανδρέας Μίγκος
Ε16. εκπαίδευση
Ε16. εκπαίδευση
Ε16. εκπαίδευση
Ε16. εκπαίδευση
Ε16. εκπαίδευση
E30. nautical pulications
Ειδικός Ναυτιλιακής κατεύθυνσης, Χαρά Κοτταρά, Αλέξανδρος Παπαδσημητρίου ΠΑΛΑΙΑ ΤΡΑΠΕΖΑ ΘΕΜΑΤΩΝ
Ε16. εκπαίδευση
Ε16. εκπαίδευση
Ε16. εκπαίδευση
Ε16. εκπαίδευση
Ε16. εκπαίδευση
E30. nautical pulications
Ε16. εκπαίδευση
Ε16. εκπαίδευση
E30. nautical pulications
Τεχνικός αυτοματισμών, Στέλιος Αντωνόπουλος, Γιώργος Νικολάου,
Ε16. εκπαίδευση
Ε16. εκπαίδευση
The Olympic mathematic marathon grades 7-12 volume 2, George Apostolopoulos, Daniel Sitaru.
Ε13. μουσική
E30. nautical pulications
Ε16. εκπαίδευση
Ε16. εκπαίδευση
Ε16. εκπαίδευση
Ε16. εκπαίδευση
E30. nautical pulications
Ε16. εκπαίδευση
Ε16. εκπαίδευση
Τέχνη Σκίτσου, εικονογραφίας και γραφικών Τεχνών, Κολίτσα Ελένη
Ε16. εκπαίδευση
Ε16. εκπαίδευση
Ε16. εκπαίδευση
Λογοτεχνική μετάφραση, διόρθωση, επιμέλεια κειμένων Μ. Λογιώτη, Δ. Ιωαννίδη, Κ. Μπαλκούρα
Ε16. εκπαίδευση
E30. nautical pulications
Ε16. εκπαίδευση
Ε16. εκπαίδευση
Στέλεχος Εμπορίας , Διαφήμισης και Προϊόντων Μάρκετινγκ, ΠΑΛΑΙΑ ΤΡΑΠΕΖΑ ΘΕΜΑΤΩΝ Χαρά Κοτταρά
Ε16. εκπαίδευση
Ε16. εκπαίδευση
E30. nautical pulications
Ο Διεθνής Κανονισμός Αποφυγής Συγκρούσεων στη Θάλασσα (Rules of the Road – ColReg) Γεώργιος Τσιμίνος
Ε.37 Διαγωνισμοί Δημ. / Κατατακτ.
Εφαρμογές Ηλεκτρονικής Διακυβέρνησης Εργασιακών (ΕΡΓΑΝΗ) και Ασφαλιστικών (ΕΦΚΑ) θεμάτων
Ε16. εκπαίδευση
ΧΗΜΕΙΑ Α΄ ΛΥΚΕΙΟΥ / ΠΡΟΣΦΟΡΑ Α΄+ Β’ ΤΟΜΟΣ. Αναστάσιος Νιώτης
Ε16. εκπαίδευση
Ε16. εκπαίδευση
Ε16. εκπαίδευση
Διδακτικοί Προσανατολισμοί / ΚΡΙΤΗΡΙΑ ΑΞΙΟΛΟΓΗΣΗΣ στη Νεοελληνική Γλώσσα και Λογοτεχνία, B’ TOMOΣ
Ε16. εκπαίδευση
Ε16. εκπαίδευση
Φυσική – Ο τρόπος σκέψης για τη Β΄ Γυμνασίου, Κώστας Θ. Ζαμπέλης, Νίκος Μπέσας, Μαρίζα Μπέσα
Ε16. εκπαίδευση
Ε16. εκπαίδευση
Ε16. εκπαίδευση
ΞΕΝΑΓΌΣ ΣΕ ΜΟΥΣΕΙΑ ΚΑΙ ΑΡΧΑΙΟΛΟΓΙΚΟΥΣ ΠΟΛΙΤΙΣΤΙΚΟΥΣ ΧΩΡΟΥΣ (ΑΤΟΜΑ ΜΕ ΠΡΌΒΛΗΜΑΤΑ ΌΡΑΣΗΣ Η ΑΚΌΗΣ)
E30. nautical pulications
Ειδικός Τουριστικου πρακτορείου, Χαρά Κοτταρά, Αρχοντη Σοπίδου, ΠΑΛΑΙΑ ΤΡΑΠΕΖΑ ΘΕΜΑΤΩΝ
Ε16. εκπαίδευση
Αρχαία Ελληνικά Β΄- Γ’ Λυκείου – Τετράδιο εργασίας, Ελένη Γιαννακουλοπούλου – Καλαβάση